If you're starting here -- I recommend that you take a few minutes and go through the Perspective Primer on this site. It introduces you to some of the jargon and fundamental topics that are used here.

Usually, when you design a shot that requires perspective, first consider if you are going to look directly into a vanishing point. Most often you will not, so now we are using at least 2 points. Consider for your shot if you can safely represent one of the 3 spatial dimensions as a set of parallel lines. If you can (when your eye level, or horizon line, is close to the level of your center of interest), then you are in a 2-point perspective application.

Consider the following 3 examples.... and, oh yeah, before I forget.....

Have fun!

Nightwing #30 Page 18

A simple application of 2-Point perspective. Note that we desire to have the viewer's eye level placed low, even a bit below the level of the panel! Thus, we will be looking above the horizon line in this image -- but we will not stray too far from the horizon line. We will force the verticals to remain parallel and use 2-point perspective.

So - let's analyze this shot:

First, find the eye level. This is your horizon line. We know that our vp's lie on this line.

Next, find the vanishing point location. Imaging the full form of the ceiling structures (that is, visualize it's complete, 3-D form in your mind). One face is significantly more revealed than the other. The face of the structure that is most revealed to us (the face that is vanishing off panel to the right) will have it's vanishing point farther away than the other face. Thus, we know that the image is not centered between the two VPs. Set the two VPs.

Last, determine the vertical (plum) line. If you were standing in the scene, and you held out a plum line, the vertical would be that line. See how it's perpendicular to the horizon line?

That's it! Then we render the detail in accordance with the system we just constructed.

Piece of cake!

Nightwing #35 Page 13

A simple application of 2-Point perspective. Note that we desire to have the viewer's eye level placed at the level of our boy, "Nite-Wing." Thus, we will be looking both above and below the horizon line in this image. We will not stray too far from the horizon line. We will force the verticals to remain parallel and use 2-point perspective.

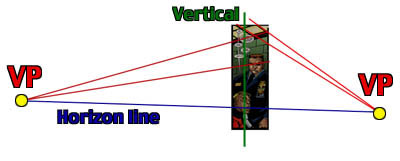
First, find the eye level. This is your horizon line. We know that our vp's lie on this line.

Next, find the vanishing point location. Imaging the full form of the cinder block just above Chief Redhorn's head (that is, visualize it's complete, 3-D form in your mind). One face is significantly more revealed than the other. The face of the block that is most revealed to us (the face that is actually showing) will have it's vanishing point farther away than the other face. Thus, we know that the image is not centered between the two VPs. Set the two VPs.

Last, determine the vertical (plum) line. If you were standing in the scene, and you held out a plum line, the vertical would be that line. See how it's perpendicular to the horizon line?

That's it! Then we render the detail in accordance with the system we just constructed.

Piece of cake!

Nightwing #38 Page 7

Though this shot looks very complicated, it reduces to a simple application of 2-point perspective. When the viewer is looking at the subject on or very near the horizon line (so that all the vertical lines are parallel), that is the clue to use 2-point perspective.

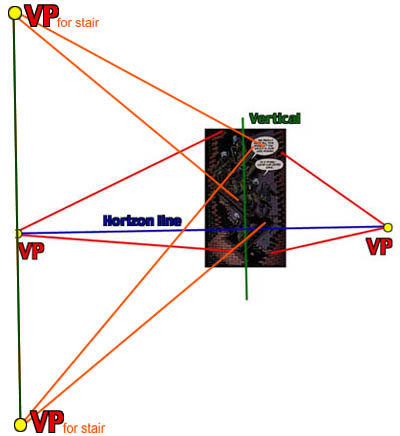
So - let's analyze this shot:

First, find the eye level. This is your horizon line. We know that our vp's lie on this line.

Next, find the vanishing point locations. Note that both faces of the outer stair wall are equally visible. Since neither face is noticably more revealed than the other, we know that our image is just about centered between the two vanishing points that lie on the horizon line! Set the vanishing points.

Next, determine the vertical (plum) line. If you were standing in the scene, and you held out a plum line, the vertical would be that line. See how it's perpendicular to the horizon line?

Last, we determine the location of the new set of VPs for the stairs. We recall that if you flatten the stair tread and riser, it is rendered with the two initial VPs. As we begin to incline the plane of the stair tread and riser, we note that the VPs begin to travel along a vertical line through the VP for that face. When you have the incline at a visually acceptable angle, set the new VP on the vertical.

When I initially figured out the perspective of this shot, I did it in a slightly different way. I first sketched the stairwell freehand. Next, I constructed a scaffolding framework (a simple vertical rectangle in perspective), and fixed the landings at each half level (simply a cross section of the vertical rectangle, at evenly spaced intervals). Last, I simply connected the proper points on the level from landing to landing. The end result is the same -- the VPs for the stair still end up on the vertical through the initial VP.

That's it! Then we render the detail in accordance with the system we just constructed.

Piece of cake!

Summary!